As we have seen in previous posts, it is essential to use alternative formulas, either time-weighted or money-weighted when there are situations of inflows or outflows in a portfolio, in order to have a proper calculation of the return.
This time, we show how to calculate the return on a money-weighted portfolio, including an example.
Formula for calculating the money-weighted return on a portfolio
The alternative formula to TWR (time-weighted return) consists of weighting by money. MWR (Money Weighted Return) is the acronym used to refer to this formula.
The most commonly used formula (by default in inbestMe) is the TWR. Alternatively and in a complementary way it can be useful to calculate the performance of our portfolio on a money-weighted basis. The MWR is useful to determine if we have been good at timing our investments.
In fact, by design, it takes into account the size of the contributions/withdrawals and the timing of the investment, which is why it is called money-weighted.
At inbestMe we prefer not to be concerned about the timing and depth of our investments. We recommend scheduling as much as possible our savings/investments on a regular and automated basis.
The MWR is calculated by finding the rate of return that will equal the present values of all cash flows to the value of the initial investment.
Those familiar with financial mathematics will see that this definition is ultimately equivalent to calculating the IRR or present value of an investment with different inflows/outflows over time.
In this case, the alternative version of the IRR is used, calculating the present value by looking for the present value of the inflows/outflows equal to 0. Note that by definition, the IRR is an annualized value.
Once obtained, we must convert it into a cumulative return with another formula.
The TWR calculation formula is, (using the acronym TWR and annualized value):
(F1): VP = 0 = CF0 + CF1/(1+TWR)^1 + CF2/(1+TWR)^2 + … CFn/(1+TWR)^n
Where CF0 is the first value deposited at the beginning of the investment and CF1, CF2, CFn are the different deposits (or withdrawals) at different times in the history of the portfolio. At the time of the calculation, we will have to consider the final value of the portfolio as one more cash flow to make the calculation.
Calculation of TWR (cumulative value):
(F2): (1+TWR)^(n)-1
Ejemplo de cómo calcular el MWR de una cartera:
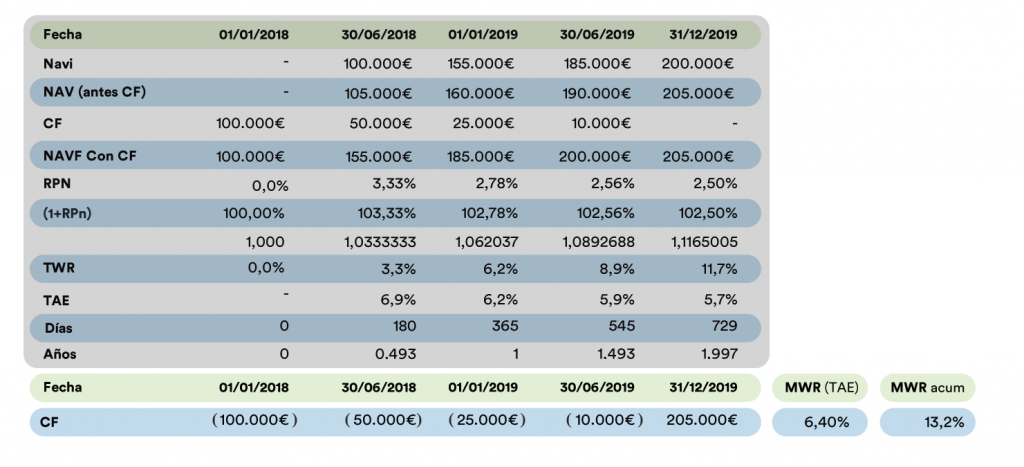
In the table above we see how to calculate the MWR (annualized first, i.e. the APR) and the cumulative value (cumulative MWR) for a portfolio starting with 100,000 euros and where there are 3 contributions of 50,000, 25,000 and 10,000 respectively.
In the table above we use the same example as for TWR. We have shaded the TWR calculation and added two more rows to see how we can extract the necessary information from the same table: to calculate the MWR we only need the dates and amounts of the cash inflows and outflows.
To make the calculation we must use an Excel with the special IRR formula, IRR.NO.PER (XIRR) specially designed for non-regular cash flows (applicable to a portfolio). We see below how in Excel we must select the values on one side and the dates on the other side.
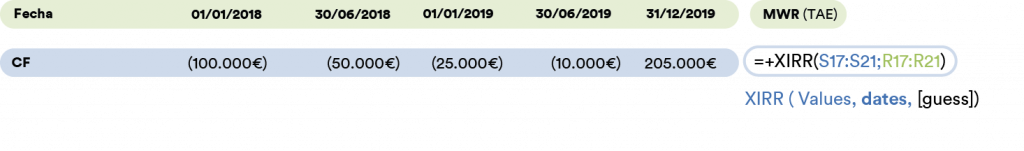
In our case, we have used the formula in English. If our Excel is in Spanish, we only have to substitute XIRR by TIR.NO.PER.
We see how the result is:
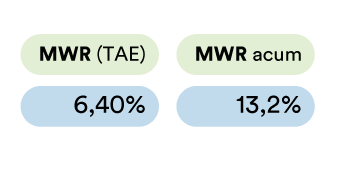
To calculate the accumulated value, we only have to apply the formula we saw before, with the numbers of this example:
(1+0.0640)^1,997 – 1 = 13,2%
Years being the period elapsed from the first investment to the calculation date (in the example: 1,997, which can be seen at the end of the example table on the right in the shaded part).
Comparison between TWR and MWR
If we compare this result with that of the TWR, we will see that in this case the resulting accumulated value is somewhat lower with the MWR formula (13.2% vs. 14.1%) and that this also obviously translates into a somewhat lower APR (6.4% vs. 6.8%).
This lower MWR would show that the investor has not got it right or has been unlucky with the timing of his investment.
On the other hand, in general, the TWR is a better measure of the manager’s success. That is why it is used more, and it is the calculation we use by default in inbestMe. Anyway, it is good to know also the MWR formula, since in extreme cases the TWR can give little relevant information for the investor.
It is important for the investor to know these calculations and the reason for their use, since by default we tend to simplify and use simple interest (a formula that is not valid when there are capital inflows and outflows).
Note: the most correct is to make these calculations after all the costs borne by the portfolio (management, trading if any and depository). This is how we do it at inbestMe by default for our clients’ portfolios and for the profitability calculation of our model portfolios. Obviously, we can always decide to make the calculation after and before costs, deducting or not from the net asset value of our portfolio.










